Pratit Luthra 4 years, 6 months ago sec 2 x=22tanx 1tan 2 x=22tanx tan 2 x2tanx1=0 (tanx1) 2 =0 tanx=1 1 Thank You ANSWER Class12th practical Shivam Mishra, Meritnation Expert added an answer, on 26/7/16 Shivam Mishra answered this Dear Student, Please find below the solution to the asked query We have f 2 tanx 1 tan 2 x = cos 2 x 1 sec 2 x 2 tanx 2 = cos 2 x 1 2 1 cos 2 x 2 sinx cosx = cos 2 x 1 2 1 2 sinx cosx cos 2 x = cos 2 x 1 2 1 sin 2 x cos 2 x = 2 cos 2 x 1 1 2 1 sin 2 x cos 2 x = 2 cos 2 x 2 1 sin 2 x cos 2 x f 2 tanx 1 tan 2 x = 1 sin 2 x We know that 2 tanx 1 tan 2 x = sin 2 x f sin 2Sin2xcos2x = 1 tan2x1 = sec2x sin 2x = 2 sin x cos x cos 2x = 2 cos2x 1 tan x = sin x cos x sec x = 1 cos x cot x = cos x sin x csc x = 1 sin x Some integration formulas R xn dx = xn1 n1 C R 1 x dx = lnjxjC R ex dx = ex C R sin x dx = cos x C R cos x dx = sin xC R
2
F(2tanx/1 tan^2x)=(cos2x 1)(sec^2x 2tanx)/2 then f(1)
F(2tanx/1 tan^2x)=(cos2x 1)(sec^2x 2tanx)/2 then f(1)-In any triangle we have 1 The sine law sin A / a = sin B / b = sin C / c 2 The cosine laws a 2 = b 2 c 2 2 b c cos A b 2 = a 2 c 2 2 a c cos B c 2 = a 2 b 2 2 a b cos C Relations Between Trigonometric FunctionsQuestion Prove The Identity Sec^2/2 Tan X = Csc 2x This problem has been solved!



2
Click here👆to get an answer to your question ️ Match the statements of Column I with values of Column II Column IColumn IIAIf f(x) = x 1 , when x2 tan^2x = 1 tan^2 x x = pi/6 {x is angle expressed in radians} General solution is x = 2* n * pi pi/6 (where n is a natural number) (tan x)× (tan 2x) = 1 or tan x = 1/ (tan 2x) =cot 2x or tan x = tan (pi/2 2x) or x = npi (pi/2–2x) or x2x= (n1/2)pi Misc 1 Misc 2 Important Misc 3 Deleted for CBSE Board 22 Exams Misc 4 Important Deleted for CBSE Board 22 Exams Misc 5 Deleted for CBSE Board 22 Exams Misc 6 Deleted for CBSE Board 22 Exams
Simply tan (AB) = tanAtanB/1tanAtanB From this formula we can derive tan (2x) as tan (xx) So tan (2x)= 2tanx/1tanxtanx We can always go forGet an answer for 'Prove the following sin 2x = (tan x)(1 cos 2x)' and find homework help for other Math questions at eNotes∫ d x cos 2 x = tan x C 2 and by combining the two constants of integration into one, we find the answer (1) ∫ ( 1 tan x) 2 d x = tan x − 2 log cos
Solve for x tan (2x)=1 tan (2x) = 1 tan ( 2 x) = 1 Take the inverse tangent of both sides of the equation to extract x x from inside the tangent 2x = arctan(1) 2 x = arctan ( 1) The exact value of arctan(1) arctan ( 1) is π 4 π 4 2x = π 4 2 x = π 4 Divide each term by 2 2Solve the equation √3 sin2x cos2x = 0 for π≤x≤π No idea how to approach this one Thanks a if sinx=7/5 and angle x is in quadrant 2 and cos y=12/13 and angle y is in quadrant 1 find sin (xy) asked in TRIGONOMETRY by harvy0496 Apprentice doubleangle



1
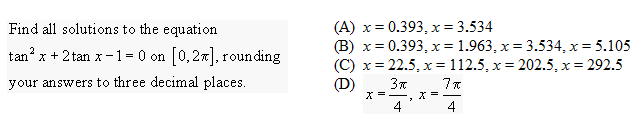



Solved Find All Solutions To The Equation Tan 2x 2tanx 1 0 Chegg Com
Rewrite sec(x) sec ( x) in terms of sines and cosines Rewrite tan(x) tan ( x) in terms of sines and cosines Multiply by the reciprocal of the fraction to divide by 1 cos(x) 1 cos ( x) Write cos(x) cos ( x) as a fraction with denominator 1 1 Cancel the common factor of cos(x) cos ( x) Explanation 2tanx 1 tan2x = 2 sinx cosx sec2x = 2 sinx cosx 1 cos2x = 2sinx cosx × cos2x 1 = 2sinxcosx Best answer We have f (2tanx/ (1 tan2x)) = 1/2 (1 cos2x) (sec2x tanx) = 1/2x 2cos2x x (1 tan2x 2tanx) = cos2x x (1 tanx)2 = {cosx x (1 tanx)}2 = (cosx sinx)2 = 1 sin (2x) Thus, f (sin 2x) = 1 sin (2x)



What Is The General Solution Of Sec 2 2x 1 Tan 2x Quora



Nwoqnovhk C Gm
Stack Exchange network consists of 178 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack ExchangeCos2 (x) (1 tan2 (x)) = 1 cos 2 ( x) ( 1 tan 2 ( x)) = 1 Replace the cos2(x) cos 2 ( x) with 1−sin2 (x) 1 sin 2 ( x) based on the sin2(x)cos2(x) = 1 sin 2 ( x) cos 2 ( x) = 1 identity 1−sin2 (x)(1tan2(x)) = 1 1 sin 2 ( x) ( 1 tan 2 ( x)) = 1 Simplify each term Tap for more stepsSolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more



Tan2x 1 ただの悪魔の画像




Tanx 4 3 X In Quadrant Ii Find The Value Of Sinx 2 Cosx 2 Tanx 2
You can put this solution on YOUR website! Solve the equation 2cos2x = √3 for 0°≤x≤360° I did this cos2x = √3 /2 2x=30 x=15 x=15, 165, 195, 345 Is this correct?Answer (1 of 4) In Trigonemetry Laws and Identities, there are some rule that we will use to prove 1 / sec² (x) = cos² (x) * tan² (x) 1 = sec² (x) * sin² (x) cos² (x) = 1 * tan (x) = sin (x) / cos (x) We will prove from the Left Hand Side We know that sec² (x) = tan² (x) 1, so 1 /




How Do You Solve Sec 2x 2tanx 0 Socratic



2tanx 1 Tan 2x 6181 2tanx 1 Tan 2x Formula
Trigonometry Solve for x tan (2x)= (2tan (x))/ (1tan (x)^2) tan(2x) = 2tan(x) 1−tan2 (x) tan ( 2 x) = 2 tan ( x) 1 tan 2 ( x) Since x x is on the right side of the equation, switch the sides so it is on the left side of the equation 2tan(x) 1− tan2(x) = tan(2x) 2 tan ( x) 1 tan 2 ( x) = tan ( 2 x)Sin(2x) = (2tan(x)) / (1tan^2(x)) *** Start with RHS 2tanx/(1tan^2x) 2tanx/(sec^2x) 2(sinx/cosx)/(1The equation `sec^2x 2*tan x = 4` has to be solved for x Using the basic identity `sin^2x cos^2x = 1` , it is possible to express sec x in terms of tan x
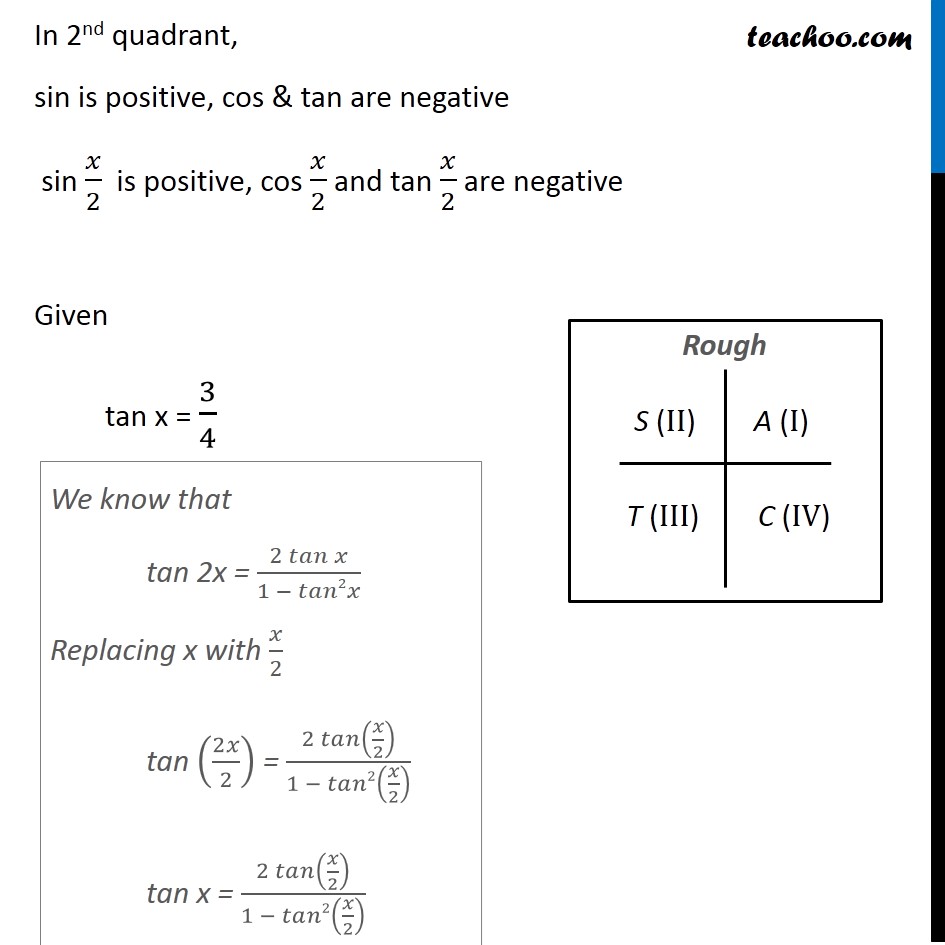



Example 28 If Tan X 3 4 Find Sin X 2 Cos X 2 Tan X 2




5 If F 2tan X 1 Tan 2 X Qquad Cos2x 1 Sec 2 X 2tan X



0 件のコメント:
コメントを投稿